Les Éléments
sur www

Euclid Elements 4.11 (Heath)
In a given circle to inscribe an equilateral and equiangular pentagon.
Let ABCDE be the given circle; thus it is required to inscribe
in the circle ABCDE an equilateral and equiangular pentagon.
Let the isosceles triangle FGH be set out having each of the
angles at G, H double of the angle at F; [[IV.
10]] 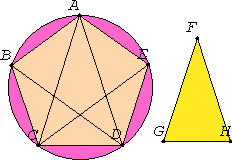 let there be inscribed in the circle ABCDE the triangle ACD
equiangular with the triangle FGH, so that the angle CAD
is equal to the angle at F and the angles at G, H
respectively equal to the angles ACD, CDA; [
let there be inscribed in the circle ABCDE the triangle ACD
equiangular with the triangle FGH, so that the angle CAD
is equal to the angle at F and the angles at G, H
respectively equal to the angles ACD, CDA; [[IV.
2]] therefore each of the angles ACD, CDA is also double
of the angle CAD.
Now let the angles ACD, CDA be bisected respectively
by the straight lines CE, DB [[I. 9]], and
let AB, BC, DE, EA be joined.
Then, since each of the angles ACD, CDA is double of
the angle CAD, and they have been bisected by the straight lines
CE,
DB,
therefore the five angles DAC, ACE,
ECD,
CDB,
BDA
are equal to one another.
But equal angles stand on equal circumferences; [[III.
26]] therefore the five circumferences AB, BC, CD,
DE,
EA
are equal to one another.
But equal circumferences are subtended by equal straight lines; [[III.
29]] therefore the five straight lines AB, BC, CD,
DE,
EA
are equal to one another; therefore the pentagonABCDE
is equilateral.
I say next that it is also equiangular.
For, since the circumference AB is equal to the circumference
DE,
let BCD be added to each; therefore the whole circumference
ABCD
is equal to the whole circumference EDCB.
And the angle AED stands on the circumference ABCD, and
the angle BAE on the circumference EDCB; therefore
the angle BAE is also equal to the angle AED. [[III.
27]]
For the same reason each of the angles ABC, BCD, CDE
is also equal to each of the angles BAE, AED; therefore
the pentagon ABCDE is equiangular.
But it was also proved equilateral; therefore in the given circle
an equilateral and equiangular pentagon
has been inscribed. Q. E. F.
Euclid Elements 4.12 (Heath)
Illustrations courtesy of David E. Joyce
About a given circle to circumscribe an equilateral and equiangular
pentagon.
Let ABCDE be the given circle; thus it is required to circumscribe
an equilateral and equiangular pentagon
about the circle ABCDE.
Let A, B, C, D, E be conceived to
be the angular points of the inscribed pentagon,
so that the circumferences AB, BC, CD, DE,
EA
are equal; [[IV. 11]] 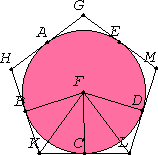 gh
A,
B,
C,
D,
E
let GH, HK,
KL,
LM,
MG be drawn touching
the circle; [
gh
A,
B,
C,
D,
E
let GH, HK,
KL,
LM,
MG be drawn touching
the circle; [[III. 16, Por.]] let the centre
F
of the circle ABCDE be taken [[III. 1]], and let
FB,
FK,
FC,
FL,
FD be joined.
Then, since the straight line KL touches the circle ABCDE
at C, and FC has been joined from the centre F to
the point of contact at C, therefore FC is perpendicular
to KL; [[III. 18]] therefore each of the angles
at C is right.
For the same reason the angles at the points B, D
are also right.
And, since the angle FCK is right, therefore the square on FK
is equal to the squares on FC, CK.
For the same reason [[I. 47]] the square on
FK
is also equal to the squares on FB,
BK; so that the squares
on FC, CK are equal to the squares on
FB,
BK,
of which the square on FC is equal to the square on FB; therefore
the square on CK which remains is equal to the square on BK.
Therefore BK is equal to CK.
And, since FB is equal to FC, and FK common,
the two sides BF, FK are equal to the two sides CF,
FK;
and the base BK equal to the base CK; therefore the angle
BFK
is equal to the angle KFC, [[I. 8]] and the angle
BKF
to the angle FKC. Therefore the angle BFC is double of the
angle KFC, and the angle BKC of the angle FKC.
For the same reason the angle CFD is also double of the
angle CFL, and the angle DLC of the angle FLC.
Now, since the circumference BC is equal to CD, the angle
BFC
is also equal to the angle CFD. [[III. 27]]
And the angle BFC is double of the angle KFC, and the
angle DFC of the angle LFC; therefore the angle KFC
is also equal to the angle LFC.
But the angle FCK is also equal to the angle FCL; therefore
FKC,
FLC
are two triangles having two angles equal to two angles and one side equal
to one side, namely FC which is common to them; therefore they will
also have the remaining sides equal to the remaining sides, and the remaining
angle to the remaining angle; [[I. 26]] therefore
the straight line KC is equal to CL, and the angle FKC
to the angle FLC.
And, since KC is equal to CL, therefore KL is double
of KC.
For the same reason it can be proved that HK is also double
of BK.
And BK is equal to KC; therefore HK is also
equal to KL.
Similarly each of the straight lines HG, GM, ML
can also be proved equal to each of the straight lines HK, KL;
therefore the pentagon GHKLM
is equilateral.
I say next that it is also equiangular.
For, since the angle FKC is equal to the angle FLC, and
the angle HKL was proved double of the angle FKC, and
the angle KLM double of the angle FLC, therefore the angle
HKL
is also equal to the angle KLM.
Similarly each of the angles KHG, HGM, GML can
also be proved equal to each of the angles HKL, KLM; therefore
the five angles GHK, HKL, KLM, LMG, MGH
are equal to one another.
Therefore the pentagon GHKLM
is equiangular.
And it was also proved equilateral; and it has been circumscribed about
the circle ABCDE. Q. E. F.
Euclid Elements 4.16 (Heath)
Illustrations courtesy of David E. Joyce
In a given circle to inscribe a fifteen-angled figure which shall be
both equilateral and equiangular.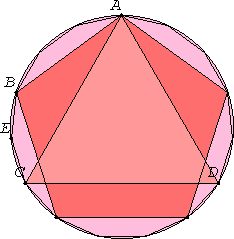
Let ABCD be the given circle;
thus it is required to inscribe in the circle ABCD a fifteenangled
figure which shall be both equilateral and equiangular.
In the circle ABCD let there be inscribed a side AC of
the equilateral triangle inscribed in it, and a side AB of an equilateral
pentagon;
therefore, of the equal segments of which there are fifteen in the
circle
ABCD,
there will be five in the circumference ABC which is one-third
of the circle,
and there will be three in the circumference AB which is one-fifth
of the circle;
therefore in the remainder BC there will be two of the equal
segments.
Let BC be bisected at E; [[III. 30]]
therefore each of the circumferences BE, EC is a fifteenth
of the circle ABCD.
If therefore we join BE, EC and fit into the circle ABCD
straight lines equal to them and in contiguity, a fifteen-angled figure
which is both equilateral and equiangular will have been inscribed in it.
Q. E. F.
And, in like manner as in the case of the pentagon,
if through the points of division on the circle we draw tangents to
the circle,
there will be circumscribed about the circle a fifteen-angled figure
which is equilateral and equiangular.
And further, by proofs similar to those in the case of the pentagon,
we can both inscribe a circle in the given fifteenangled figure and
circumscribe one about it. Q. E. F.
Euclid Elements 6.20 (Heath)
Illustrations courtesy of David E. Joyce
Similar polygons are divided into similar triangles, and into
triangles equal in multitude and in the same ratio as the wholes, and
the polygon has to the polygon a ratio duplicate of that which the corresponding
side has to the corresponding side.
Let ABCDE, FGHKL be similar polygons, and let AB
correspond to FG; I say that the polygons ABCDE, FGHKL
are divided into similar triangles, and into triangles equal in multitude
and in the same ratio as the wholes, and the polygon ABCDE has to
the polygon FGHKL a ratio duplicate of that which AB has
to FG.
Let BE, EC, GL, LH be joined. 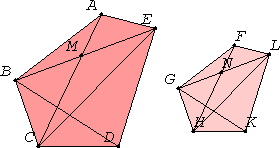
Now, since the polygon ABCDE is similar to the polygon FGHKL,
the angle BAE is equal to the angle GFL; and, as BA
is to AE, so is GF to FL. [[VI. Def.
1]]
Since then ABE, FGL are two triangles having one angle
equal to one angle and the sides about the equal angles proportional, therefore
the triangle ABE is equiangular with the triangle FGL; [[VI.
6]] so that it is also similar; [[VI. 4 and Def.
1]] therefore the angle ABE is equal to the angle FGL.
But the whole angle ABC is also equal to the whole angle FGH
because of the similarity of the polygons; therefore the remaining angle
EBC
is equal to the angle LGH.
And, since, because of the similarity of the triangles ABE,
FGL,
as EB is to BA, so is LG to GF, and moreover
also, because of the similarity of the polygons, as AB is
to BC, so is FG to GH, therefore, ex aequali, as EB
is to BC, so is LG to
GH; [[V. 22]]
that is, the sides about the equal angles EBC,
LGH are proportional;
therefore the triangle
EBC is equiangular with the triangle LGH,
[[VI. 6]] so that the triangle EBC is also
similar to the triangle
LGH. [[VI. 4 and Def. 1]]
For the same reason the triangle ECD is also similar to the triangle
LHK.
Therefore the similar polygons ABCDE, FGHKL have been
divided into similar triangles, and into triangles equal in multitude.
I say that they are also in the same ratio as the wholes, that is,
in such manner that the triangles are proportional, and ABE, EBC,
ECD
are antecedents, while FGL, LGH, LHK are their consequents,
and that the polygon ABCDE has to the polygon
FGHKL a ratio
duplicate of that which the corresponding side has to the corresponding
side, that is AB to FG.
For let AC, FH be joined.
Then since, because of the similarity of the polygons, the angle ABC
is equal to the angle FGH, and, as AB is to BC, so
is FG to GH, the triangle ABC is equiangular
with the triangle FGH; [[VI. 6]] therefore the
angle BAC is equal to the angle GFH, and the angle BCA
to the angle GHF.
And, since the angle BAM is equal to the angle GFN, and
the angle ABM is also equal to the angle FGN, therefore the
remaining angle AMB is also equal to the remaining angle FNG;
[[I. 32]] therefore the triangle ABM is equiangular
with the triangle FGN.
Similarly we can prove that the triangle BMC is also equiangular
with the triangle GNH.
Therefore, proportionally, as AM is to MB, so is FN
to NG, and, as BM is to MC, so is GN to NH;
so that, in addition, ex aequali, as AM is to MC, so
is FN to NH.
But, as AM is to MC, so is the triangle ABM to
MBC,
and AME to EMC; for they are to one another as their bases.
[[VI. 1]]
Therefore also, as one of the antecedents is to one of the consequents,
so are all the antecedents to all the consequents; [[V. 12]]
therefore, as the triangle AMB is to BMC, so is ABE
to CBE.
But, as AMB is to BMC, so is AM to MC;
therefore also, as AM is to MC, so is the triangle ABE
to the triangle EBC.
For the same reason also, as FN is to NH, so is the triangle
FGL
to the triangle GLH.
And, as AM is to MC, so is FN to NH; therefore
also, as the triangle ABE is to the triangle BEC, so is the
triangle FGL to the triangle GLH; and, alternately, as the
triangle ABE is to the triangle FGL, so is the triangle BEC
to the triangle GLH.
Similarly we can prove, if BD, GK be joined, that, as
the triangle BEC is to the triangle LGH, so also is the triangle
ECD
to the triangle LHK.
And since, as the triangle ABE is to the triangle FGL,
so is EBC to LGH, and further ECD to LHK, therefore
also, as one of the antecedents is to one of the consequents so are all
the antecedents to all the consequents; [[V. 12] therefore,
as the triangle ABE is to the triangle FGL, so is the polygon
ABCDE
to the polygon FGHKL.
But the triangle ABE has to the triangle FGL a ratio
duplicate of that which the corresponding side AB has to the corresponding
side FG; for similar triangles are in the duplicate ratio of the
corresponding sides. [[VI. 19]]
Therefore the polygon ABCDE also has to the polygon FGHKL
a ratio duplicate of that which the corresponding side AB has to
the corresponding side FG.
Therefore etc.
Euclid Elements 6.29 (Heath)
Illustrations courtesy of David E. Joyce
To a given straight line to apply a parallelogram equal to a given rectilineal
figure and exceeding by a parallelogrammic figure similar to a given one.
Let AB be the given straight line, C the given rectilineal
figure to which the figure to be applied to AB is required to be
equal, and D that to which the excess is required to be similar;
thus it is required to apply to the straight line AB a parallelogram
equal to the rectilineal figure C and exceeding by a parallelogrammic
figure similar to D. 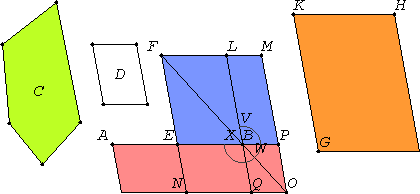
Let AB be bisected at E; let there be described on EB
the parallelogram BF similar and similarly situated to D;
and let GH be constructed at once equal to the sum of BF,
C
and similar and similarly situated to D. [[VI. 25]]
Let KH correspond to FL and KG to FE.
Now, since GH is greater than FB, therefore KH
is also greater than FL, and KG than FE.
Let FL, FE be produced, let FLM be equal to KH,
and FEN to KG, and let MN be completed; therefore
MN
is both equal and similar to GH.
But GH is similar to EL; therefore MN is
also similar to EL; [[VI. 21]] therefore EL
is about the same diameter with MN. [[VI. 26]]
Let their diameter FO be drawn, and let the figure be described.
Since GH is equal to EL, C, while GH is
equal to MN, therefore MN is also equal to EL, C.
Let EL be subtracted from each; therefore the remainder,
the gnomon XWV, is equal to C.
Now, since AE is equal to EB, AN is also equal
to NB [[I. 36]], that is, to LP [[I.
43]].
Let EO be added to each; therefore the whole AO
is equal to the gnomon VWX.
But the gnomon VWX is equal to C; therefore AO
is also equal to C.
Therefore to the given straight line AB there has been applied
the parallelogram AO equal to the given rectilineal figure C
and exceeding by a parallelogrammic figure QP which is similar to
D,
since PQ is also similar to EL [[VI. 24]].
Q. E. F.
Euclid Elements 12.1 (Heath)
Illustrations courtesy of David E. Joyce
Similar polygons inscribed in circles are to one another as the squares
on the diameters.
Let ABC, FGH be circles, let ABCDE, FGHKL
be similar polygons inscribed in them, and let BM, GN be
diameters of the circles; I say that, as the square on BM is to
the square on GN, so is the polygon ABCDE to the polygon
FGHKL. 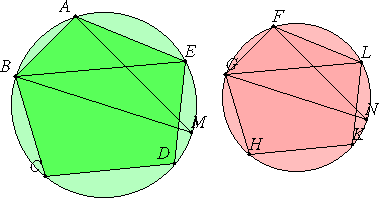
For let BE, AM, GL, FN be joined.
Now, since the polygon ABCDE is similar to the polygon FGHKL,
the angle BAE is equal to the angle GFL, and, as BA
is to AE, so is GF to FL. [VI. Def. I]
Thus BAE, GFL are two triangles which have one angle
equal to one angle, namely the angle BAE to the angle GFL,
and the sides about the equal angles proportional; therefore the triangle
ABE
is equiangular with the triangle FGL. [[VI. 6]]
Therefore the angle AEB is equal to the angle FLG.
But the angle AEB is equal to the angle AMB, for they
stand on the same circumference; [[III. 27]] and the
angle FLG to the angle FNG; therefore the angle AMB
is also equal to the angle FNG.
But the right angle BAM is also equal to the right angle GFN;
[[III. 31]] therefore the remaining angle is equal to
the remaining angle. [[I. 32]]
Therefore the triangle ABM is equiangular with the triangle
FGN.
Therefore, proportionally, as BM is to GN, so is BA
to GF. [[VI. 4]]
But the ratio of the square on BM to the square on GN
is duplicate of the ratio of BM to GN, and the ratio of the
polygon ABCDE to the polygon FGHKL is duplicate of the ratio
of BA to GF; [[VI. 20]] therefore also,
as the square on BM is to the square on GN, so is the polygon
ABCDE
to the polygon FGHKL.
Therefore etc. Q. E. D.
Euclid Elements 12.6 (Heath)
Illustrations courtesy of David E. Joyce
Pyramids which are of the same height and have polygonal bases are to
one another as the bases.
Let there be pyramids of the same height of which the polygons ABCDE,
FGHKL
are the bases and the points M, N the vertices; I say that,
as the base ABCDE is to the base FGHKL, so is the pyramid
ABCDEM
to the pyramid FGHKLN. 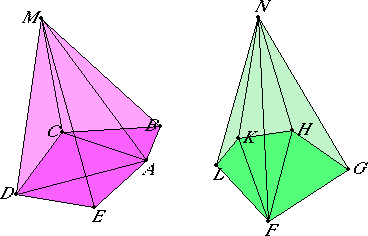
For let AC, AD, FH, FK be joined.
Since then ABCM, ACDM are two pyramids which have triangular
bases and equal height, they are to one another as the bases; [[XII.
5]] therefore, as the base ABC is to the base ACD, so
is the pyramid ABCM to the pyramid ACDM.
And, componendo, as the base ABCD is to the base ACD,
so is the pyramid ABCDM to the pyramid ACDM. [[V.
18]]
But also, as the base ACD is to the base ADE, so is the
pyramid ACDM to the pyramid ADEM. [[XII. 5]]
Therefore, ex aequali, as the base ABCD is to the base ADE,
so is the pyramid ABCDM to the pyramid ADEM. [[V.
22]]
And again componendo, as the base ABCDE is to the base ADE,
so is the pyramid ABCDEM to the pyramid ADEM. [[V.
18]]
Similarly also it can be proved that, as the base FGHKL is to
the base FGH, so is the pyramid FGHKLN to the pyramid FGHN.
And, since ADEM, FGHN are two pyramids which have triangular
bases and equal height, therefore, as the base ADE is to the base
FGH,
so is the pyramid ADEM to the pyramid FGHN. [[XII.
5]]
But, as the base ADE is to the base ABCDE, so was the
pyramid ADEM to the pyramid ABCDEM.
Therefore also, ex aequali, as the base ABCDE is to the base
FGH,
so is the pyramid ABCDEM to the pyramid FGHN. [[V.
22]]
But further, as the base FGH is to the base FGHKL, so
also was the pyramid FGHN to the pyramid FGHKLN.
Therefore also, ex aequali, as the base ABCDE is to the
base FGHKL, so is the pyramid ABCDEM to the pyramid FGHKLN.
[[V. 22]] Q. E. D.
Euclid Elements 13.7 (Heath)
Illustrations courtesy of David E. Joyce
If three angles of an equilateral pentagon,
taken either in order or not in order, be equal, the pentagon
will be equiangular.
For in the equilateral pentagon
ABCDE let, first, three angles taken in order, those at A,
B, C, be equal to one another; I say that the pentagonABCDE
is equiangular. 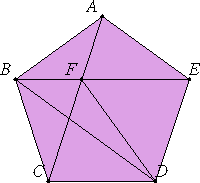
For let AC, BE, FD be joined.
Now, since the two sides CB, BA are equal to the two
sides BA, AE respectively, and the angle CBA is equal
to the angle BAE, therefore the base AC is equal to the base
BE,
the triangle ABC is equal to the triangle ABE, and the remaining
angles will be equal to the remaining angles, namely those which the equal
sides subtend, [[I. 4]] that is, the angle BCA
to the angle BEA, and the angle ABE to the angle CAB;
hence the side AF is also equal to the side BF. [[I.
6]]
But the whole AC was also proved equal to the whole BE;
therefore the remainder FC is also equal to the remainder FE.
But CD is also equal to DE.
Therefore the two sides FC, CD are equal to the two sides
FE,
ED;
and the base FD is common to them; therefore the angle
FCD
is equal to the angle FED. [[I. 8]]
But the angle BCA was also proved equal to the angle AEB;
therefore the whole angle BCD is also equal to the whole angle AED.
But, by hypothesis, the angle BCD is equal to the angles at
A,
B;
therefore the angle AED is also equal to the angles at
A,
B.
Similarly we can prove that the angle CDE is also equal to the
angles at A, B, C; therefore the pentagonABCDE
is equiangular.
Next, let the given equal angles not be angles taken in order, but
let the angles at the points A, C, D be equal; I say
that in this case too the pentagon
ABCDE is equiangular.
For let BD be joined.
Then, since the two sides BA, AE are equal to the two
sides BC, CD, and they contain equal angles, therefore the
base BE is equal to the base BD, the triangle ABE
is equal to the triangle BCD, and the remaining angles will be equal
to the remaining angles, namely those which the equal sides subtend; [[I.
4]] therefore the angle AEB is equal to the angle CDB.
But the angle BED is also equal to the angle BDE, since
the side BE is also equal to the side BD. [[I.
5]]
Therefore the whole angle AED is equal to the whole angle CDE.
But the angle CDE is, by hypothesis, equal to the angles at
A,
C;
therefore the angle AED is also equal to the angles at
A,
C.
For the same reason the angle ABC is also equal to the angles
at A, C, D.
Therefore the pentagon ABCDE
is equiangular. Q. E. D.
Go to page: 1; 2