Hyperbolic Tessellations
David E. Joyce (djoyce@clarku.edu)
The hyperbolic plane has more space
in it then the Euclidean plane. There are only three regular tessellations
of the Euclidean plane, namely {3,6} in which equilateral triangles meet
six at each vertex, {4,4} in which squares meet four at each vertex, and
{6,3} in which hexagons meet three at each vertex. But the hyperbolic plane
can be tiled by any kind of regular polygon, and in many different ways.
If
1/n + 1/k < 1/2,
then {n,k} is a tessellation of the hyperbolic plane by
regular n-gons where k of them meet at each vertex. So, for
instance, {5,4} is a tiling of the hyperbolic plane by pentagons meeting
four at each vertex.
(A notation like {3,6} is called a Schläfli
symbol.)
The Poincare Disk
The hyperbolic plane can not be metrically
represented in the Euclidean plane, but Poincare described ways that it
can be conformally represented in the Euclidean plane. One of those is
to represent the hyperbolic plane as the points inside a disk. For this
representation, a straight line in the hyperbolic plane is represented
as the part (in the disk) of a circle that meets the boundary of the disk
at right angles. What this means will be clear in the examples displayed
below.
The regular tessellation {5,4} of the hyperbolic plane
A regular tessellation is a covering of
the plane by regular polygons so that the same number of polygons meet
at each vertex. For instance, here is a representation of the tessellation
of the hyperbolic plane by pentagons where four pentagons meet at each
vertex, that is, the {5,4}-tessellation.
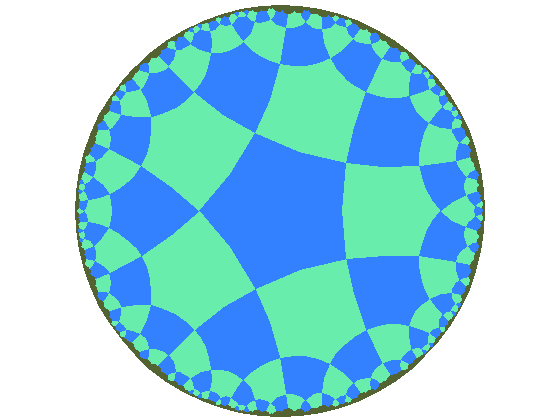
It may look like the sides of the pentagons are curved, but that's just
because of the representation we're using. In the actual hyperbolic plane
they would be straight. Also, the pentagon in the middle looks larger,
but, again, that's due to the representation. You just can't put an infinite
plane in a finite region without a lot of distortion.
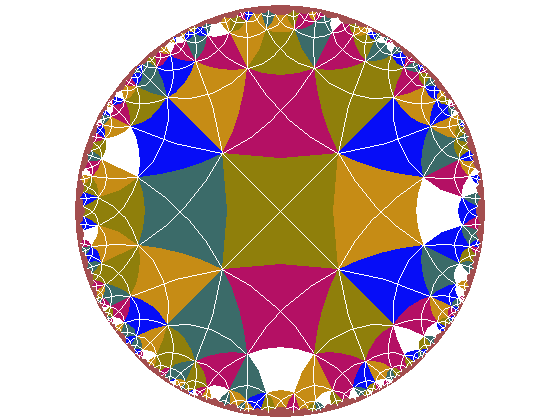
The dual tessellation {4,5} of the hyperbolic plane
For a dual tessellation you reverse the
roles of the faces and the vertices.
The dual of a {5,4} tessellation is a {4,5} tessellation, that is, a tiling
by squares, five squares meeting at each vertex. (Here, "square" means
regular quadrilateral, a four-sided figure with the same angle at each
vertex. It doesn't mean the corners are right-angled, so maybe "square"
isn't the best term.) For this picture, the diagonals are drawn so you
can see the straight lines that go off to infinity.
Some quasiregular tessellations of the hyperbolic plane
A quasiregular tessellation is built
from two kinds of regular polygons so that two of each meet at each vertex,
alternately. We'll use the notation quasi-{n,k} to denote
a quasiregular tessellation by n-gons and k-gons.
Every regular tessellation {n,k}
gives rise to a quasiregular tessellation quasi-{n,k} by
connecting the midpoints of the edges of the regular tessellation. In the
Euclidean plane there are just two quasiregular tessellations: quasi-{3,6}
arises from both {3,6} and {6,3}, while quasi-{4,4} comes from {4,4}. (Of
course, quasi-{n,n} is the same as {n,4}.)
Since there are more regular tessellations
of the hyperbolic plane than of the Euclidean plane, there are more quasiregular
tessellations, too. Here are some of them. First a quasi-{5,4} tessellation.
The pentagons are in red or yellow while the squares are in orange. It
looks like a plaid disk.

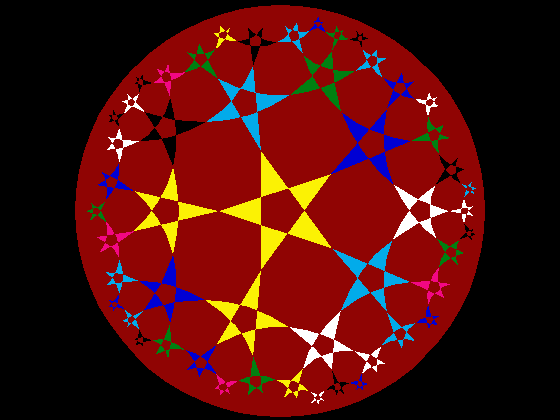
Here's a variation of it where the squares aren't colored, but pentagrams
of various colors are placed in the pentagons (the pentagons don't show,
either).
A quasi-{3,7} tessellation is built
of triangles and heptagons. In the next picture, the triangles are colored
a variety of colors while the heptagons are left black.

David E. Joyce
August, 1994 Subject:
Re: Euclid's
Elements
Date:
Mon, 19 Oct
1998 15:58:24 -0400
From:
djoyce@aleph0.clarku.edu
(Dave Joyce)
To:
david.Boeno@wanadoo.fr
Dear David Boeno,
I'm very sorry for not replying earlier.
Of course links are welcome, as mentioned on the copyright
page.
Furthermore, if you have any need for any image, please
feel
free to copy it.
Sincerely
Dave Joyce
Clark University
p.s. I couldn't get anything on the web at perso.wanadoo.fr today.